Tools

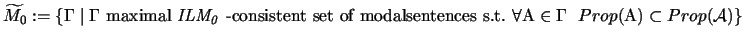
Definition 5.1   $\widetilde{M_0}:= \{ \Gamma \mid \Gamma \mbox{ maximal $\mathit{ILM_0}$ -consis... ...orall \mathrm{A} \in \Gamma \ \ Prop( \mathrm{A}) \subset Prop( \mathcal{A}) \}$. Prop(B) is the set of propositional variables occurring in B.

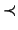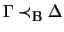The definitions of $\prec$ and $\Gamma \prec_{\mbox{\footnotesize {B}}} \Delta$ are precisely as before.

In perfect analogy with the case of $\mathit{ILM}$ we have the following two lemmas:

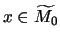
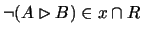
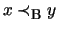
Lemma 5.2   Let $x \in \widetilde{M_0}$ and $\neg ( A \rhd B ) \in x \cap R$. There exists y such that $x \prec_{\mbox{\footnotesize {B}}} y$and $A \in y$. Moreover, y can be chosen to contain a ``maximal amount'' of $\Box$-formulas.

Lemma 5.3  

Let $x \in \widetilde{M_0}$ with $A \rhd B \in x$ and let $y \in \widetilde{M_0}$ be such that $x \prec_{\mbox{\footnotesize {C}}} y$ and $A \in y$. There exists $z \ _C \! \succ x$ , with $B \in z$.

The M₀-axiom is essentially used only in the next lemma.

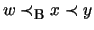
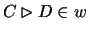
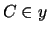
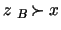
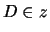
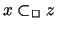
Lemma 5.4   Consider $w \prec_{\mbox{\footnotesize {B}}} x \prec y$, all in $\widetilde{M_0}$, such that $C \rhd D \in w$, and $C \in y$. Then there exists $z \ _B \! \succ x$ with both $D \in z$ and $x \subset_{\Box} z$. This z can be chosen to be maximal w.r.t. the $\Box$-inclusion.